Vector problems are a common way to solve issues involving quantities like acceleration, momentum, force, velocity, and displacement. But first, let's understand the difference between scalars and vectors. Scalars are quantities without direction, like kilograms or centimeters. For example, your weight and height are scalar quantities, expressed in terms of an amount and a unit. Other examples of scalars include speed, mass, temperature, energy, length, and distance. Vectors, on the other hand, have both magnitude and direction. For instance, the momentum of an object is equal to its mass per acceleration and has a direction, making it a vector unit. Examples of vector quantities are velocity, acceleration, momentum, displacement, and force, including weight. If you understand the difference between scalars and vectors, you'll be able to solve a variety of vector problems.

Resolving vectors into components

When dealing with complex vector problems, resolving vectors into components is a useful technique. To do this, we measure the horizontal and vertical length of the vector and state them as two separate magnitudes. Let's look at an example to understand this better. Suppose we want to find the components of the vector shown below. We can begin by determining its horizontal and vertical lengths. In this case, the horizontal length is 12 and the vertical length is 10. The magnitudes of the components of the vector are the lengths we have measured. We always get one horizontal value and one vertical value when we resolve a vector into its components. In this case, the components of the vector are two separate vectors, one horizontal and one vertical, with magnitudes of 12 and 10, respectively. But what if we can't measure the horizontal and vertical lengths of the vector? Don't worry - we can still resolve the vector into its components. Let's learn how to do it.

‍

‍

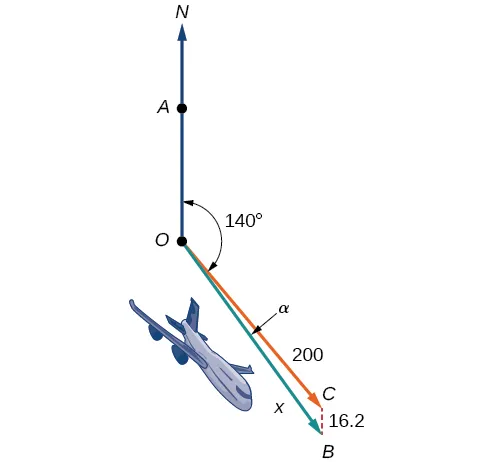
Knowing a vector's gradient angle allows us to determine the magnitudes of its horizontal and vertical components. In the case of the vector v above, let's say the gradient angle is a. Using trigonometry, we can establish the relationship between the angle and magnitude of the components. Let's determine the magnitude of the horizontal component vx. We know that:

vx = v * cos(a)

Solving the equation for vx, we get:

vx = v * cos(a)

Similarly, let's determine the magnitude of the vertical component vy. We know that:

vy = v * sin(a)

Solving the equation for vy, we get:

vy = v * sin(a)

By calculating the magnitudes of the horizontal and vertical components of a vector, we can resolve it into its components and solve complex vector problems.

Adding vectors together

Adding two vectors together is called finding their resultant. There are two methods for adding vectors together: using scale diagrams or using trigonometry. Let's first discuss the method of using scale diagrams. To find the resultant vector using this method, we draw a scale diagram of the vectors we want to add, connecting them tip-to-tail. The following example will illustrate this concept:

Suppose a man initially walks northeast for 11.40 meters, then continues to walk east for 6.6 meters, and finally walks northwest for 21.26 meters before stopping. We need to determine the total displacement of the man. To do this, we need to represent the lengths he walked as vectors, each with the correct direction and magnitude. Let's name his first movement as vector A, his second as vector B, and his third as vector C. We can draw these vectors as arrows on a diagram, with the tail of each vector starting from the tip of the previous vector. Then, we can draw a vector from the starting point of vector A to the end point of vector C, representing the total displacement of the man. We can measure the magnitude and direction of this resultant vector using a ruler and a protractor. This is how we can find the resultant vector using scale diagrams.

‍

‍

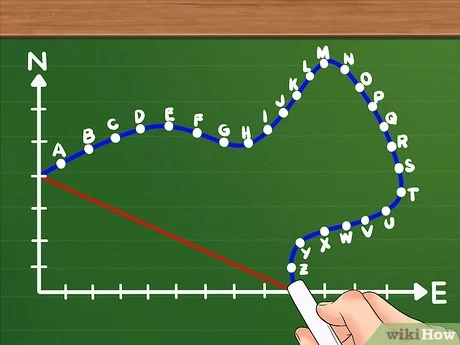
If you measure the total displacement with a ruler, you will see that it is 23.094 metres in the northern direction, even though the man walked for 39.26 metres. Let’s prove this mathematically by resolving the vectors into their components. In this particular example, we only need the vertical components since the total displacement is only vertical.

‍

To determine Ay, we apply the equation for resolving vectors into their components:

We don’t have to determine the components of B, as this example doesn’t include a vertical component. For determining Cy, we apply the same equation.The total displacement is the sum of Ay and Cy, which can be calculated as follows:

Determining the resultant vectors by using trigonometry

If two vectors are perpendicular to each other, we can find the resultant using trigonometry. Let’s again look at an example.

Two friends are pushing a box. The two forces they apply are perpendicular to each other. One of the friends is applying a force of 3 Newtons (F1) in the eastern direction, while the other is applying a force of 4 Newtons (F2) in the northern direction. Determine the resultant vector for the total force that is being applied to the box.

‍

Two forces, F1  and F2, are perpendicular to each other, which means that the magnitude of Ftotal is equal to the hypotenuse of the triangle formed by these vectors.

Vector Problems - Key takeaways Physics uses vectors to express any quantity that has a direction and magnitude. To resolve a vector into its components, we need to measure the horizontal and vertical lengths of the vector and express them as two separate vectors. To add vectors together, we can use scale diagrams or trigonometry. To determine the resultant vectors by using scale diagrams, we need to connect the vectors ‘tip-to-tail’. If two vectors are perpendicular to each other, we can find the resultant using the Pythagorean theorem.

Vector Problems

How can we solve vector problems?

To solve vector problems, we apply one of two techniques for adding up vectors. One uses scale diagrams, while the other applies trigonometry. For complex vector problems, it is essential to know how to resolve vectors into their components.

How can we solve a unit vector problem?

In order to solve a unit vector problem, we need to divide the vector by its magnitude.

How can we solve vector word problems?

In order to solve vector word problems, we need to take the given variables and draw a scale diagram, while putting the variables in the right places.

‍