In our daily lives, we tend to use words like distance, speed, and acceleration without really understanding the difference between them But to physicists, these terms are classified as either scalar or vector quantities. Scalar quantities only have a magnitude or size, like time or energy, with no direction associated with them. On the other hand, vector quantities have both a magnitude and a direction, like force or acceleration. It's important to note that all vector quantities are associated with a specific direction. So, next time you hear these terms, remember that they can be either scalar or vector!

Scalars and vectors: meaning and examples

Let's take a closer look at some examples to help us better understand scalars and vectors.

For instance, imagine you have a box and you move it 5 meters. This distance is an example of a scalar quantity because it only has magnitude, or size, associated with it. There is no direction associated with the distance you moved the box.

Now, imagine you push the box with a force of 10 newtons to the right. This force is an example of a vector quantity because it has both magnitude (10 newtons) and direction (to the right).

Another example of a vector quantity is velocity. If you're driving in a car and you're going 50 kilometers per hour to the north, your velocity is a vector quantity because it has both magnitude (50 kilometers per hour) and direction (north).

Acceleration is another vector quantity. For example, if you're in a car and you step on the gas pedal, you're causing the car to accelerate. Acceleration has both magnitude (how fast the car is speeding up) and direction (in the direction the car is moving).

So next time you hear these terms, try to identify whether they're scalar or vector quantities based on whether they have only magnitude or both magnitude and direction.

If you tell somebody that the distance between points A and B is 5 meters, you are talking about a scalar quantity because you are not specifying any direction. Five meters is just a magnitude (distance), and the direction could be any. So, distance is a scalar quantity.

However, if you tell someone you moved the box 5 meters to the right (east), as depicted in figure 1, you are now talking about a vector quantity. Why? Because you have now specified a direction associated with the movement. And in physics, this is referred to as displacement. Hence, displacement is a vector quantity.

Now let’s say it took you 2 seconds to move the box to the right.

‍

If you were to calculate how quickly you moved the box, you are calculating the speed of the movement. In the above example, the speed is:

The speed is a scalar quantity as it does not have any direction.

However, if you say the box moved with a speed of 2.5m/s to the right, this becomes a vector quantity. The speed with a direction is velocity, and a change in velocity is, in turn, known as acceleration (m/s2), which is also a vector quantity.

Mass and weight: which one is a scalar and a vector quantity?

The mass and weight of a body may seem the same, but they are not.

Mass: The quantitative measure of inertia of a body, which is the tendency of a body to resist the force that can cause a change in its speed or position. Mass has an SI unit of kilograms.

Weight: The gravitational pull acting on a mass. It has an SI unit of Newtons.

Mass does not have any direction, and it will be the same no matter where you are in the universe! So we can categorise mass as a scalar quantity.

Weight, on the other hand, is the force acting on an object, and since force has a direction, weight is a vector quantity.

Another way to look at this is if you place one object on Earth and another object with the same mass on the Moon. Both objects will have the same mass but a different weight due to the gravitational pull on the Moon (1.62 m/s2), which is smaller compared to Earth.

How can we represent vectors?

We can represent vectors with an arrow, as shown below.

‍

The length depicts the magnitude, the tail is the initial point of a vector, the sense of a vector is given by the order of two points on a line parallel to the vector, and the orientation tells you at which angle the vector is pointing. The combination of orientation and sense specify the direction of the vector.

Vector examples: how can we perform vector addition?

Let’s look at some examples of how to perform vector addition.

Say you have two vectors of 10N and 15N, and both are pointing towards the east. The sum of these vectors becomes 25N towards the east.

‍

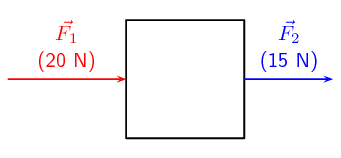
Now, if we change the direction of the 15N towards the west (-15 N), the resultant vector becomes -5 N (pointing towards the west). A vector quantity can have positive and negative signs. The sign of a vector shows that the direction of the vector is the opposite of the reference direction (which is arbitrary).

‍

Now, of course, all vector additions are not as straightforward as shown above. What would you do if the two vectors were perpendicular to each other? This is where we need to improvise a little bit.

Head-to-tail rule

With this rule, we can calculate the resultant vector by joining the tail of the first vector with the head of the second vector. Take a look at the figures below.

‍

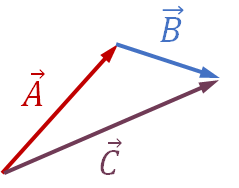
A vector force of 30N acts in the east direction, while a vector force of 40N acts in the north direction. We can calculate the resultant vector by joining the tail of the 30N vector with the head of the 40N vector. The vectors are perpendicular, so we can use the Pythagorean theorem to solve the resultant vector as shown in figure 7.

‍

With a bit of trigonometry and applying the Pythagorean theorem, the resultant vector becomes 50N. Now, as we discussed, a vector quantity has a magnitude as well as a direction, so we can calculate the angle of the 50N vector by using an inverse tangent of 40/30 (perpendicular/base). The angle is then 53.1° from the horizontal for the above example.

Resolving a vector into its components

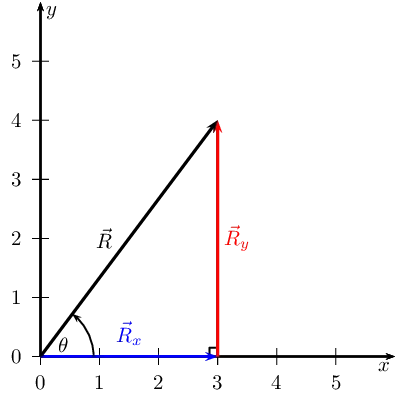
Using the same example from above, what if we only had the 50N vector force with an angle from the horizontal and were asked to find its horizontal and vertical components?

Splitting a single vector into two or more vectors that produce a similar effect to the original vector is called the resolution of vectors.

Let’s have a look at an example to explain this concept further.

Suppose a vector force F of 150N is applied at an angle of 30 degrees from the surface.

‍

We can split the vector F into a horizontal component (Fx) and a vertical (Fy) component as depicted below:

‍

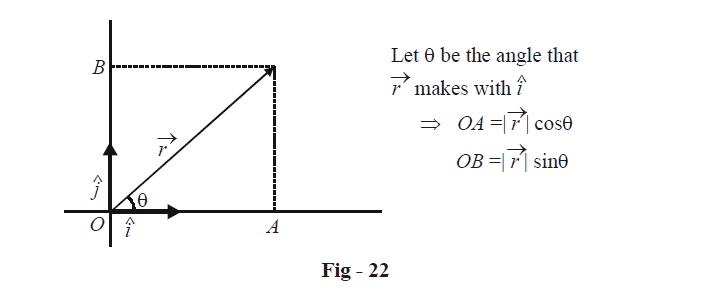
Calculating Fx and Fy by using trigonometry gives us:

Resolving components of a force on an inclined plane

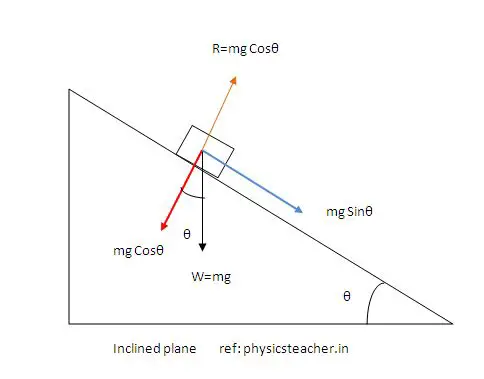
As you might have figured out by now, calculations in physics are never this straightforward! Not every surface is horizontal – sometimes surfaces may be at an incline, and you have to calculate and resolve components along an inclined plane.

‍

Figure 10 shows a box on a surface at an angle θ from the horizontal. The weight of the box, mg, is acting downwards with a mass m and the gravitational pull g.

If we split the mg vector into the horizontal and vertical components,

the vertical component will be perpendicular to the inclined surface, andthe horizontal component of mg will be parallel to the inclined surface.

‍

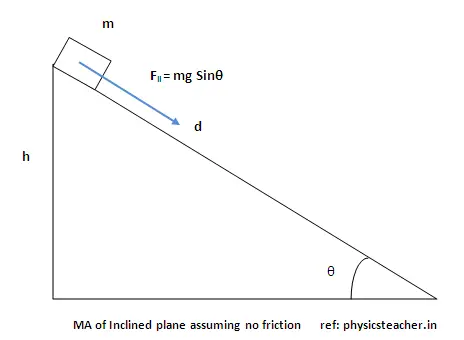
The θ angle between the mg and mgcosθ will be the same as the inclined surface angle from the horizontal. The force that will accelerate the box down the slope will be mgsinθ (Fg), and the reaction force Fn (from Newton’s third law) will be equal to mgcosθ. Hence,

‍

Equilibrium of coplanar force systems

If forces are acting on a body and the body is stationary or moving with a constant velocity (not accelerating), such an instance is called equilibrium. The lines of forces must pass through the same point for an object to be in equilibrium.

In the diagram below, a uniform ladder is leaning against a smooth wall (no friction). The ladder’s weight acts downwards, and the normal reaction force acts at an angle of 90° from the wall.

‍

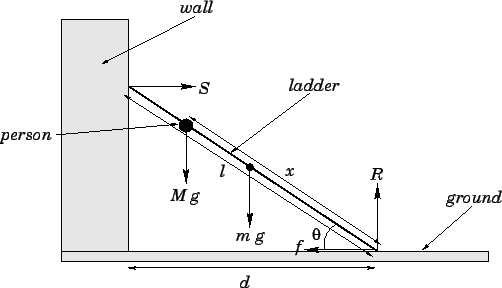
If you extend these forces, you will see that they cross at a certain point. Because the object is in equilibrium, the force from the ground must also pass through the same point as the other forces do.

‍

By resolving the force from the ground into its vertical and horizontal components, the normal reaction force from the ground acts upwards, and the friction force from the ground acts along the surface.

‍

To understand how forces work, it's important to know about scalars and vectors. A scalar quantity only has magnitude, like time or distance. But a vector quantity has both magnitude and direction, and can be represented with an arrow.

When two forces are equal in magnitude and opposite in direction, they cancel each other out. This is called equilibrium. For example, if you have a ladder leaning against a wall, the normal force from the wall (right force) is equal to the frictional force acting along the ground (left force), and the weight of the ladder (downward force) is equal to the reaction force from the ground (upward force).

To find the resultant vector, you add vectors in the same direction and subtract vectors in opposite directions. You can calculate the resultant vector of two vectors using the head-to-tail rule, and the resultant vector of perpendicular vectors using the Pythagorean theorem.

If a vector is at an angle to the horizontal or vertical, you can resolve it into its x and y components. And to achieve equilibrium, the line of forces must intersect at a common point and cancel each other out.

In summary, understanding scalars and vectors is crucial to understanding how forces work and how they can be balanced to achieve equilibrium.

Scalar and Vector

What is the difference between a scalar and a vector?

The difference between a scalar and a vector is that scalar quantities have a magnitude only, whereas vector quantities have a magnitude as well as a direction. 

What is a scalar and a vector?

A scalar quantity is a quantity with a magnitude (size) only. A vector quantity is a quantity that has both a magnitude and a direction associated with it. 

Is force a vector or a scalar?

Force is a vector quantity.

Is power a vector?

No, power is not a vector quantity. It is a scalar quantity. 

Is speed a vector or a scalar?

Speed is a scalar quantity. Velocity is a vector quantity.

‍