Astronomy is a cool branch of science because it studies stuff that doesn't happen on Earth. To study space, we need special tools called telescopes. These tools are also known as astronomical telescopes.

Astronomical telescopes have been around for a really long time. In the 17th century, Galileo Galilei was the first person to point a telescope up at the sky. His telescope could make things look twenty times closer, which helped him see faraway things with his own eyes. Nowadays, we don't need to use our eyes to look at space. We have digital astronomical telescopes that can collect data really accurately. Plus, if we only use our eyes, we can only see things in the visible part of the spectrum.

Even though we have fancy telescopes now, it's still important to study the original telescopes that Galileo made. This helps us understand how all telescopes work, even the ones with super high magnification.

How do lenses work?

To understand how telescopes let us see faraway things up close, we first need to know how lenses work. Lenses are physical devices that can focus or spread out light by bending it. Every lens has a focal distance, which is the distance at which an object needs to be placed in order to form a clear image that looks like it's at an infinite distance. There are two main types of lenses: converging and diverging. Converging lenses focus light towards a point, while diverging lenses spread it out. For our purposes, we're only going to focus on converging lenses since those are used to build the simplest kind of astronomical telescope.

We'll look at how converging lenses work in a diagram and also explain the math behind them so we can understand how telescopes can magnify things.

Diagrams of converging lenses

See the two images below:

‍

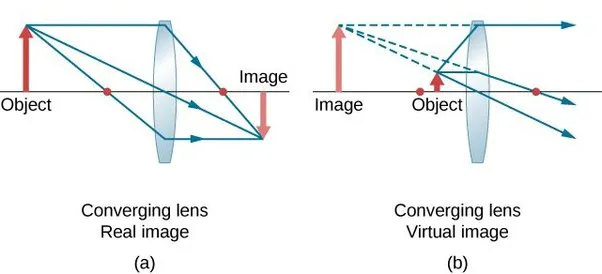
To create a diagram of the possible images formed with a converging lens, follow these simple steps:

1. Draw the object on one side of the lens (it doesn't matter which side, but we chose the left).
2. Draw the lens and mark its focal distance as "f." The distances don't need to be exact, but they should be proportional to the real distances.
3. A ray travels horizontally from the object to the lens and is refracted by the lens, then travels to the focal point on the line4 A ray point without being refracted.
   5 If the don't cross on the right side of the lens, extend them to the left with a dashed line to form a virtual image.

By following these steps, we can find the two rays that make up the undotted lines in the diagrams above. It's important to note that the position of the object with respect to the focal point of the lens determines how the image is formed.

If the object is beyond the focal point, the light rays meet on the other side of the lens and form an image that is upside down. The image will be bigger if the object is placed at less than twice the focal distance, and smaller if it's beyond that distance.

If the object is between the focal point and the lens, the undotted light rays don't meet on the other side of the lens. By continuing the lines with dotted lines, we can see that the image is formed on the same side of the lens as the object, and it's always bigger than the object.

If the object is on the focal point, the image is formed on the other side of the lens at an infinite distance with the same size.

Finally, when an image of an object is formed on the opposite side of a lens, it can be projected onto a screen and is called a "real image." When an image is formed on the same side of a lens, it can't be projected and is called a "virtual image."

Mathematical description of converging lenses

The equation for converging lenses is:

1/f = 1/xo + 1/xi

where xo is the distance of the object to the lens, f is the focal distance of the lens, and xi is the distance of the image of the object. If xi is negative, it will be a virtual image, and if xi is positive, it will be a real image.

The magnification of lenses (the amount of growth of the image of an object with respect to its actual size) follows this equation:

|yi|/|yo| = |xi|/|xo|

where yi is the vertical size of the image, yo is the vertical size of the object, and the absolute value appears because we take magnification to be a positive quantity. The absolute value indicates the amount of growth, and the sign indicates whether the orientation of the image is the same as the object’s or if it is the opposite.

Let's take a converging lens with a focal distance f of 10cm and calculate the characteristics of the image of an object placed at different distances:

a) If the object is placed at 15cm from the lens:
Using the formula for converging lenses, we have:

1/f = 1/xo + 1/xi
1/10 = 1/15 + 1/xi
xi = -30cm

Since the quantity is negative, the image is formed on the same side of the lens and upside down (as we know from the first diagram). We can now calculate the magnification:

|yi|/|yo| = |xi|/|xo|
|yi|/|yo| = |-30|/|15|
|yi|/|yo| = 2

This means that the image produced is double the size of the object.

b) If the object is placed at 30cm from the lens:
Using the formula for converging lenses, we have:

1/f = 1/xo + 1/xi
1/10 = 1/30 + 1/xi
xi = 15cm

Since the quantity is positive, the image is formed on the opposite side of the lens and upside down. We can calculate the magnification:

|yi|/|yo| = |xi|/|xo|
|yi|/|yo| = |15|/|30|
|yi|/|yo| = 0.5

This means that the image produced is half the size of the object.

c) If the object is placed at 5cm from the lens:
Using the formula for converging lenses, we have:

1/f = 1/xo + 1/xi
1/10 = 1/5 + 1/xi
xi = 3.33cm

Since the quantity is negative, the image is formed on the same side of the lens and upright (as we know from the second diagram). We can calculate the magnification:

|yi|/|yo| = |xi|/|xo|
|yi|/|yo| = |-3.33|/|5|
|yi|/|yo| = 0.666

This means that the image produced is

What is an astronomical refracting telescope?

Refracting telescopes consist of two lenses: an objective lens and an eyepiece lens. The objective lens is a converging lens that collects light from a distant object and forms a real image at the focal point of the lens. This image is inverted, but it is magnified and can be viewed through the eyepiece lens.

The eyepiece lens is also a converging lens that magnifies the real image produced by the objective lens. The magnification of the telescope is given by the ratio of the focal lengths of the two lenses.

Magnification = focal length of objective lens / focal length of eyepiece lens

The resolving power of a telescope is determined by the size of the objective lens. The larger the lens, the greater the amount of light it can collect and the sharper the image it can produce. However, the size of the objective lens is limited by the size of the telescope itself.

The field of view of a telescope is determined by the eyepiece lens. The larger the eyepiece lens, the wider the field of view. However, larger eyepieces also reduce the amount of light reaching the eye, so there is a trade-off between field of view and brightness.

The aperture of a telescope is the diameter of the objective lens. This determines the amount of light that the telescope can collect and therefore its brightness. The larger the aperture, the brighter the image. However, larger apertures also increase the cost and size of the telescope.

In summary, telescopes use the combined refraction of two lenses to collect and magnify light from distant objects. The size of the objective lens determines the resolving power, the size of the eyepiece lens determines the field of view, and the aperture determines the brightness of the image.

Definition and characteristics of an astronomical telescope of two lenses

The magnification of a two-lens astronomical refracting telescope is given by the ratio of the focal lengths of the objective lens and the eyepiece lens:

Magnification = fo/fe

where fo is the focal length of the objective lens and fe is the focal length of the eyepiece lens.

The resolving power of the telescope is determined by the size of the objective lens. The larger the lens, the greater the amount of light it can collect and the sharper the image it can produce. However, the size of the objective lens is limited by the size of the telescope itself.

The aperture of the telescope is the diameter of the objective lens. This determines the amount of light that the telescope can collect and therefore its brightness. The larger the aperture, the brighter the image. However, larger apertures also increase the cost and size of the telescope.

The field of view of the telescope is determined by the eyepiece lens. The larger the eyepiece lens, the wider the field of view. However, larger eyepieces also reduce the amount of light reaching the eye, so there is a trade-off between field of view and brightness.

In summary, a two-lens astronomical refracting telescope uses two converging lenses to magnify the images of distant objects. The objective lens collects the incident light and creates an image, while the eyepiece lens magnifies the image and creates a virtual image at infinity so that it can be seen by an observer. The magnification is given by the ratio of the focal lengths of the lenses, and the resolving power and aperture are determined by the size of the objective lens. The field of view is determined by the size of the eyepiece lens.

Astronomical telescope magnification and formula derivation

Finally, let’s investigate how powerful astronomical telescopes are. For a telescope, the usual calculations with lenses do not yield helpful information because we are working with objects so far away that we can consider their light rays to be parallel. Furthermore, we are also working with images that are placed exactly at the focal point of a lens.

As we only offer a simplified version of lenses in the first sections, we apply these general ideas to obtain the magnification. Since magnification is the ratio of the size of the image to the actual object, we can obtain this information by using the angles α and β. It turns out that for astronomical measurements, these angles have the following expressions:

To find the ratio of the sizes of the image of the object and the image we observe, we have to divide the two angles, which yields:

We can now see why it is useful that the objective lens has a very large focal distance and the eyepiece lens has a small focal distance.

If we have a telescope whose objective lens has a focal distance of 1m and whose eyepiece lens has a focal distance of 1mm, the magnification is 1000. This is the power of telescopes, and they can be made of more complex combinations of lenses to increase their power even.

‍

Astronomical Telescopes - Key takeaways An astronomical telescope is a device that allows us to gather data on very distant objects in the universe. Optical telescopes are based on the refractive properties of lenses. Galileo Galilei created the first optical telescope. The most basic model of a telescope is made of two converging lenses combined in a specific way. The magnification power is the amount of growth of the image observed with respect to the received image. The whole functioning of lens-based refracting telescopes is based on the laws of optics that govern how lenses deviate light rays.

Images

Refracting telescopes – telescopes using large lenses for their objective. https://www.schoolphysics.co.uk/age16-19/Optics/Optical%20instruments/text/Telescopes_/index.html

Astronomical Telescopes

Do astronomers use reflecting or refracting telescopes?

Astronomers use both kinds of telescopes since they allow us to gather data. However, the resolution and power of reflecting telescopes are greater, so they are the ones used for science. 

How does an astronomical telescope work?

An astronomical telescope receives light and processes it via a set of lenses and/or mirrors to magnify it and process it with digital or visual means.

What is an astronomical telescope?

An astronomical telescope is a device that allows us to gather data on distant objects in the universe.

‍